where you start learning everything about electrical engineering computing, electronics devices, mathematics, hardware devices and much more.
What is Singular Value Decomposition? In this article we have discussed about Implementation of SVD ins Machine Learning. SVD, singular value decomposition is one of the methods of dimension reduction. In the field of machine learning, dimension reduction has become Read More …
How to find the basis of a vector space? How to find the basis of a vector space V? In order to find the basis of a vector space , we need to check two properties: The vectors should be Read More …
Introduction to Linear system of equations How to solve system of linear equations in Linear Algebra? A linear equation in n variables x1,x2,x3,…..xn is an equation that can be written in the form of where b and coefficients are real Read More …
Introduction to eigen values and eigen vectors How to Find the Eigen Values and Eigen Vectors? explain with examples Eigen Values and Eigen Vectors has a great significance in many engineering problems specially in case of linear systems where we Read More …
Introduction Use of MATLAB Software for Linear Algebra. With a focus on complex arithmetic problem and the unambiguous concept of line algebra, this paper discusses the introduction of MATLAB software in algebra teaching and describes the practical steps and results Read More …
Since, by applying head to tail rule on the vectors components, we can generate the original vector A. After that we need to find the projections of one vector to another vector. This projection is subtracted from the other vector and hence these two vectors become orthogonal.
So, for two vectors A and B lets say we want to generate a new set of vectors W and Y that will be orthogonal to each other. We will assume
Introduction Gauss Elimination Vs Gauss Jordan Elimination Methods for Solving System of Linear Equations. In this article we examine the comparisons between the Gauss and Gauss-Jordon methods for fixing system of linear equations. It was very unusual by solving the Read More …
Introduction The fast Fourier transform method and ill-conditioned matrices. We have studied the problem of calculating numerical solutions of the linear algebra equation, a*x=b, where a denotes an N*N ill-conditioned coefficient matrix. It is known that Gaussian elimination methods linked Read More …
Introduction What are the Applications of Matrices in Cryptography? The science of encoding and decoding signals is known as cryptography. Cryptography is widely used in everyday life to protect tactful data such as affinity card numbers. This analysis investigates matrices Read More …
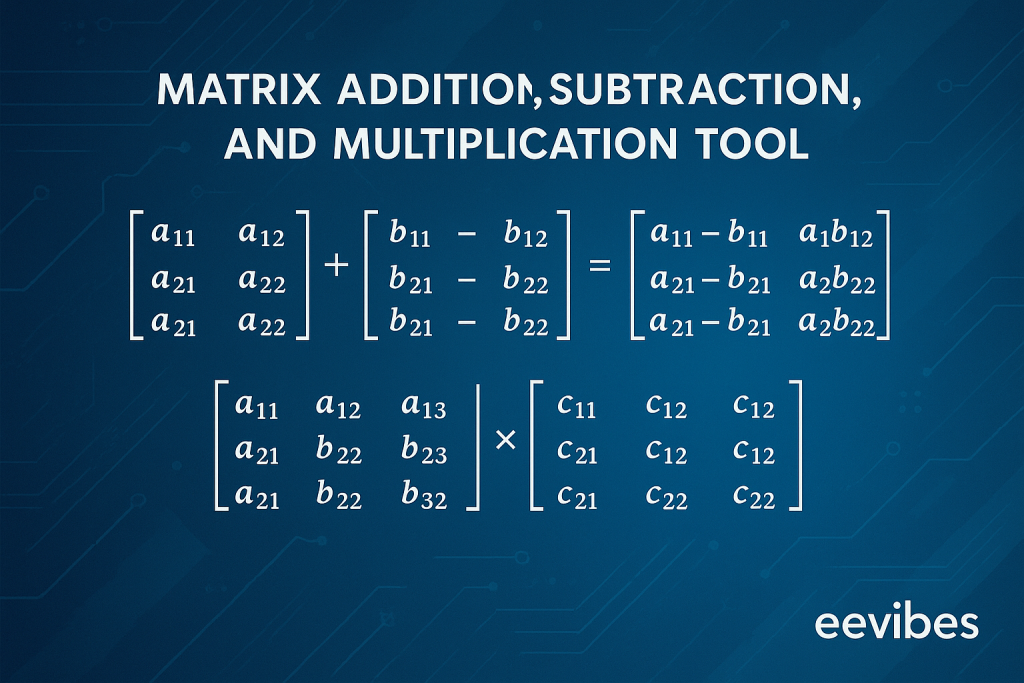
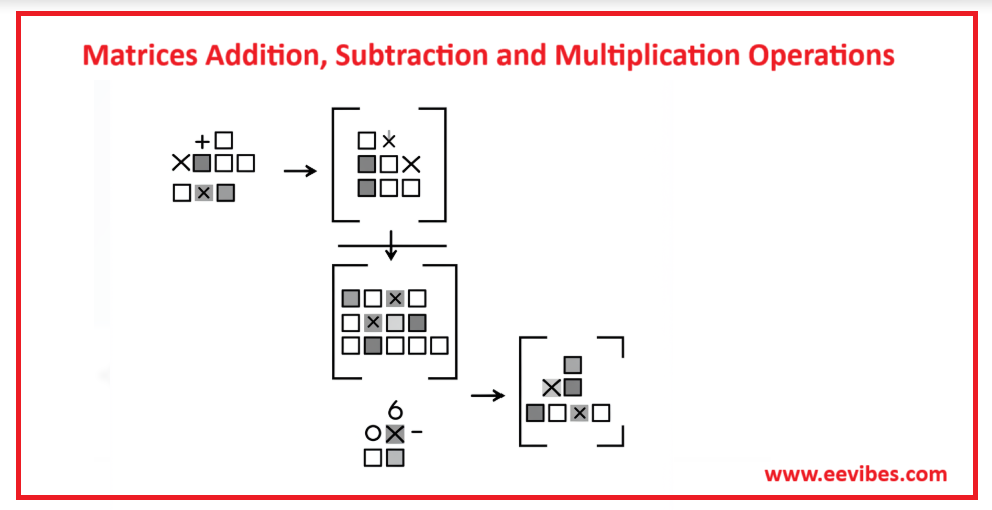
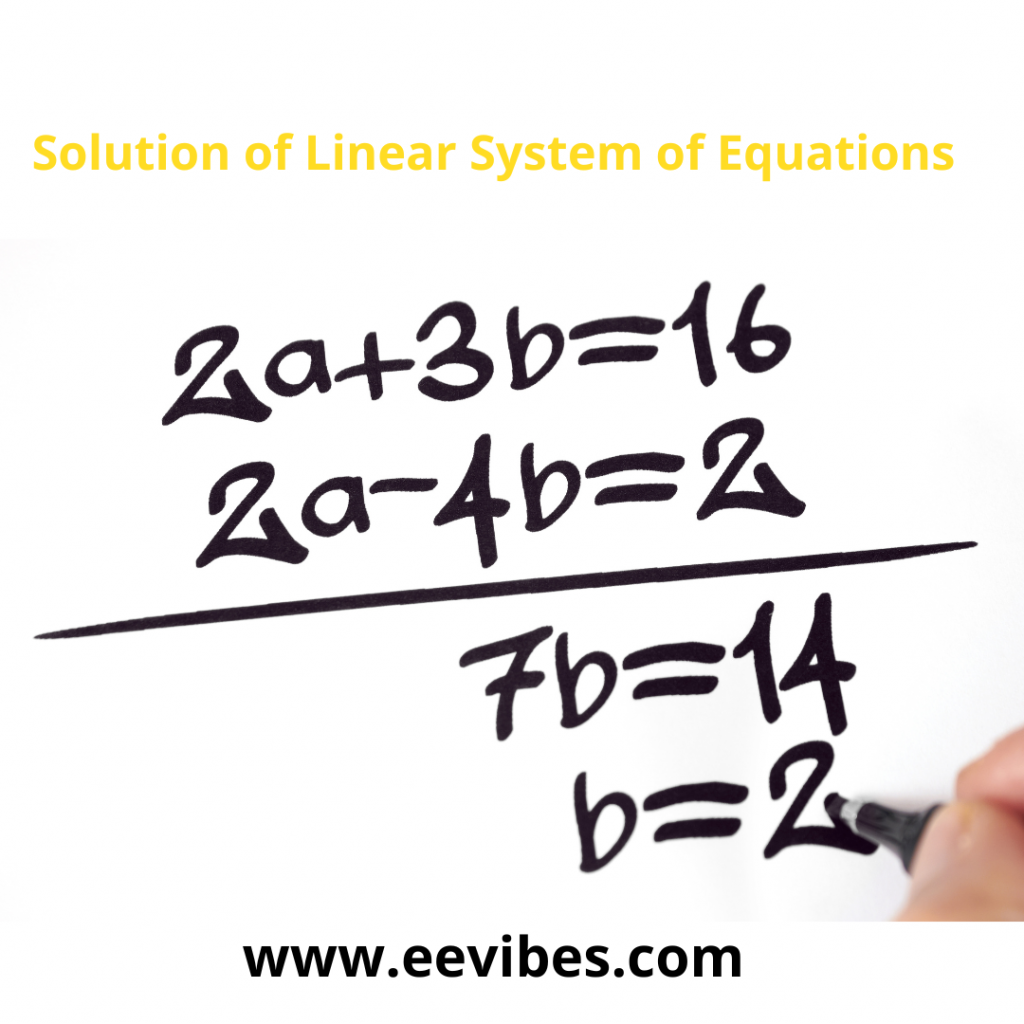
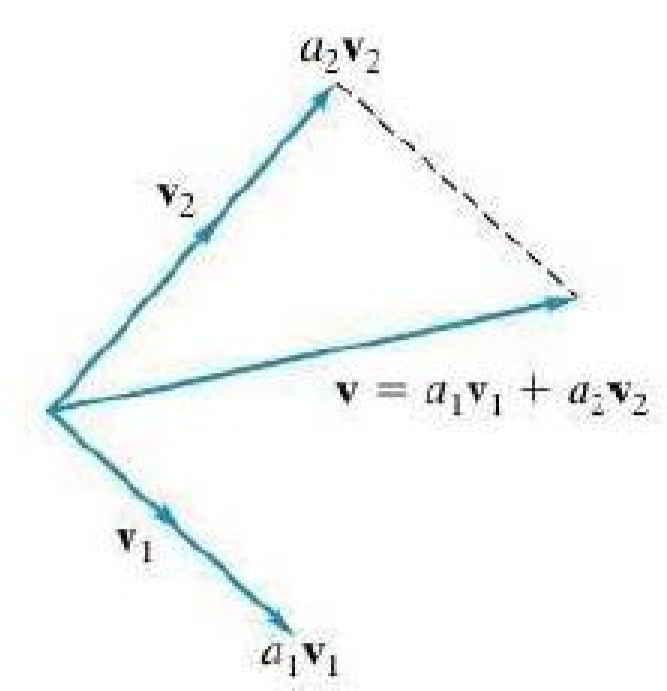
Introduction Power Method and Inverse Power Method for Eigen Values and Eigen Vectors. When looking for the greatest Eigen-pair, the power approach is usually used. The Inverse Power approach, on the other hand, is used to determine the smallest Eigen-pair. Read More …